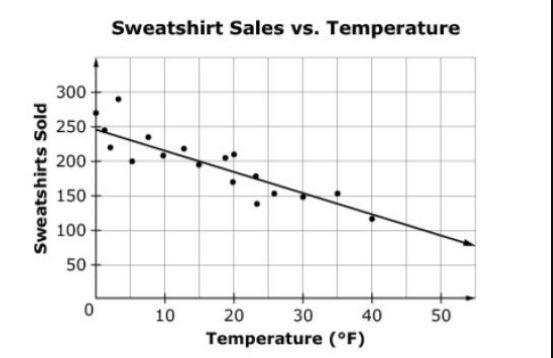
How many sweatshirts sold is BEST predicted with an outside temperature of 5 °F? A) 170 B) 200 C) 230 D) 250

The median value in the data set is the average of the two middle values which is: 23.

In an ordered data set, the median is the middle value or average of the two middle values in the data set.

List out each data point in the data set given:

20, 20, 20, 22, 22, 24, 26, 26, 26, 26

The middle values in the data set are, 22 and 24.

Median = (22 + 24)/2

Median = 23

Learn more about the median on:

https://brainly.com/question/26177250

Answer:

20 and 13

Step-by-step explanation:

If one list is 'a' and the other one is 'b' then

a-b = 7 and a+b = 33

a-b+a+b = 7+33

2a = 40

a = 20

Now, 20 - b = 7

-b = -13

b = 13

Answer:

Choice D is the correct answer

Step-by-step explanation:

Use the foil method and expand

Answer:

it is a

Step-by-step explanation:

because 11 +1 8n binary is qlways 100 bbecause 11 is in the 4th in the truth table of binary numbers and 100 is in the 5th so 11 + 1 = 4+1

100 = 5

pls mark it as the brainlyest answer

Answer:

It's a.

Step-by-step explanation:

11 binary = 2 + 1 = 3 in decimal.

So 11 + 1 = 3 + 1 = 4 in decimal.

and 4 in binary = 100  (2^2 + 0 + 0)

Answer:

I'm so confused. I don't get the question. I know the options are:

A) 3,4, 5

B) 2, 10, 4

C) 2, 10, 3

D) 2, 15, 2

but I don't understand the question. Could you maybe be a bit more specific?

Answer:

As Per Provided Information

An ellipse has a vertex at (0, −7), a co-vertex at (4, 0), and a center at the origin (0,0) .

We have been asked to find the equation of the ellipse in standard form .

As we know the standard equation of an ellipse with centre at the origin (0,0). Since its vertex is on y-axis

[tex] \underline\purple{\boxed{\bf \: \dfrac{ {y}^{2} }{ {a}^{2} } \: + \: \dfrac{ {x}^{2} }{ {b}^{2} } = \: 1}}[/tex]

where,

Substituting these values in the above equation and let's solve it

[tex] \qquad\sf \longrightarrow \: \dfrac{ {y}^{2} }{ {( - 7)}^{2} } \: + \dfrac{ {x}^{2} }{ {(4)}^{2} } = 1 \\ \\ \\ \qquad\sf \longrightarrow \: \dfrac{ {y}^{2} }{49} \: + \frac{ {x}^{2} }{16} = 1 \\ \\ \\ \qquad\sf \longrightarrow \: \: \dfrac{ {x}^{2} }{16} \: + \dfrac{ {y}^{2} }{49} = 1[/tex]

Therefore,

So, your answer is 2nd Picture.

Answer:

maximum height is 45/4 in feet

The maximum height of the ball is 45/4 in feet

The unitary method is a method for solving a problem by the first value of a single unit and then finding the value by multiplying the single value.

Given tha Pam kicked a soccer ball down the field. Part of the ball's path, including its highest point, is described by the function below, where f(x) is the height of the ball in feet.

F(x)= 12x^2+2x        

Thus for this quadratic , the max value will occur at x= -b/2a

=  - 2 / (2 x 1/12) = -12

Plug in -12 to find the max height

= (-12)^2 + 2 x-12

=  45/4 in feet

Learn more about the unitary method, please visit the link given below;

https://brainly.com/question/23423168

#SPJ2

Answer:

6 .x = 121°

Sum of all interior angles of a 7 sided polygon is 900°.

Answer:

c = 292; h = 225

c = cheeseburgers

h = hamburgers

Step-by-step explanation:

2 Equations;

h + c = 517

c = h + 67

h + h + 67 = 517  |  Substitution

2h + 67 = 517 | Subtract on Both Sides

2h = 450  | Divide

h = 225

Plug the h value in the second equation to find the value of c.

c = h +67 | Original Equation

c = 225 + 67 | Substitution

c = 292

Answer:

y = 10

rate of change = 5

Step-by-step explanation:

The initial value is the beginning output value, i.e. the y-value when x = 0

Therefore, the initial value is y = 10

rate of change = change in y ÷ change in x

⇒ rate of change = (40 - 30) ÷ (6 - 4)

                            = 10 ÷ 2

                            = 5

take two points : (0, 10), (2, 20)

rate of change:

[tex]\rightarrow \sf \dfrac{y_2-y_1}{x_2-x_1}[/tex]

[tex]\rightarrow \sf \dfrac{20-10}{2-0}[/tex]

[tex]\rightarrow \sf 5[/tex]

equation :

y - 10 = 5 ( x - 0 )

y = 5(x) + 10

so initial value:

y = 5(0) + 10

y = 10

Answer:

-9/25

Step-by-step explanation:

-1^10 = -1

since (-1)(-1)(-1)(-1)(-1)(-1)(-1)(-1)(-1)(-1) = -1

Anything to the power of 0 is 1 so -22^0 = 1

Now our equation is -1 + 1 -(3/5)^2

Simplifying this:

0 -9/25 =

-9/25

Answer:

in fraction: 41/25

= 1.64

Step-by-step explanation:

[tex](-1)^{10} =1[/tex]

[tex](-22)^{0} =1[/tex]

[tex](\frac{3}{5} )^{2} =\frac{9}{25}[/tex]

Then

[tex]1+1-\frac{9}{25} =2-\frac{9}{25} =\frac{50}{25} -\frac{9}{25} =\frac{41}{25}[/tex]

decimal: 1.64

Hope this helps

Solution :-

Here, we have been given that lines f and g are parallel. Thus, the angle measuring 135° and ∠2 are vertically opposite angles.

And we know that vertically opposite angles measure same. Thus,

∠2 = 135°

And,

∠2 + ∠6 = 180° ( Co - interior angles sum up to 180° )

135° + ∠6 = 180°

∠6 = 180° - 135°

∠6 = 45°

Now,

we see that ∠6 and ∠5 are making a linear pair of angles, and we know that angles in a linear pair measure 180° Thus,

∠5 + 45° = 180°

∠5 = 180° - 45°

∠5 = 135°

Thus, the value of angle 5 is 135°

Hope that helps. :)

Answer:

nonlinear

Step-by-step explanation:

Part B: nonlinear

The radius increases so the line is an arc going upwards not a linear line.

In other words, not a straight line.

PLEASE RATE!! I hope this helps!!

If you have any questions comment below

Answer:

9/8 or 1 1/8

Step-by-step explanation:

3*3/8

3/1*3/8

numerator times numerator and denominator times denominator:

3×3/1*8 = 9/8 or 1 1/8

Answer:

30

Step-by-step explanation:

The distance between 2 points on a number line uses the following equation:

point2 - point1.

In this case we get 15 - (-15) which equals 30.

Answer:

  7 m, 24 m, 25 m

Step-by-step explanation:

This problem can be solved by writing an equation expressing the given relationships and the Pythagorean theorem. Or, it can be solved by reference to common Pythagorean triples. Here, we're interested in a triple that has a difference of 1 between the hypotenuse and the longer leg. Such triples include:

  {3, 4, 5}, {5, 12, 13}, {7, 24, 25}, {9, 40, 41}, {11, 60, 61}, ...

We note that for the triple {7, 24, 25}, the longer leg is 3 more than 3 times the shorter leg: 3 +3(7) = 24.

The side lengths are:

__

In case you're unfamiliar with Pythagorean triples, or you want to write the equation, you can let s represent the length of the shorter side. Then the longer side is (3s+3) and the hypotenuse is (3s+4). The Pythagorean theorem tells you the relation is ...

  (3s +4)² = (3s +3)² +s²

  9s² +24s +16 = 9s² +18s +9 +s²

  s² -6s -7 = 0 . . . . . subtract the left side and put in standard form

  (s -7)(s +1) = 0 . . . . factor

  s = 7  or  -1 . . . . . . solutions to the equation

The side length must be positive, so the shorter leg is 7 meters long. Then the other two legs are ...

  3s +3 = 3(7) +3 = 24 . . . . meters

  3s +4 = 3(7) +4 = 25 . . . . meters

The side lengths are 7 m, 24 m, and 25 m.

Answer:

See below ↓

Step-by-step explanation:

We have a graph given to us.

⇒ Take the points to form an equation!

The points

Making an equation

Take two points

Slope:-

[tex]\\ \rm\rightarrowtail m=\dfrac{6-1}{4+2}=\dfrac{5}{6}[/tex]

Equation in point slope form

[tex]\\ \rm\rightarrowtail y-1=5/6(x+2)[/tex]

[tex]\\ \rm\rightarrowtail 6y-6=5x+10[/tex]

[tex]\\ \rm\rightarrowtail 6y=5x+16[/tex]

[tex]\\ \rm\rightarrowtail y=5/6x+8/3[/tex]

Recall the geometric sum,

[tex]\displaystyle \sum_{k=0}^{n-1} x^k = \frac{1-x^k}{1-x}[/tex]

It follows that

[tex]1 - x + x^2 - x^3 + \cdots + x^{2020} = \dfrac{1 + x^{2021}}{1 + x}[/tex]

So, we can rewrite the integral as

[tex]\displaystyle \int_0^\infty \frac{x^2 + 1}{x^4 + x^2 + 1} \frac{\ln(1 + x^{2021}) - \ln(1 + x)}{\ln(x)} \, dx[/tex]

Split up the integral at x = 1, and consider the latter integral,

[tex]\displaystyle \int_1^\infty \frac{x^2 + 1}{x^4 + x^2 + 1} \frac{\ln(1 + x^{2021}) - \ln(1 + x)}{\ln(x)} \, dx[/tex]

Substitute [tex]x\to\frac1x[/tex] to get

[tex]\displaystyle \int_0^1 \frac{\frac1{x^2} + 1}{\frac1{x^4} + \frac1{x^2} + 1} \frac{\ln\left(1 + \frac1{x^{2021}}\right) - \ln\left(1 + \frac1x\right)}{\ln\left(\frac1x\right)} \, \frac{dx}{x^2}[/tex]

Rewrite the logarithms to expand the integral as

[tex]\displaystyle - \int_0^1 \frac{1+x^2}{1+x^2+x^4} \frac{\ln(x^{2021}+1) - \ln(x^{2021}) - \ln(x+1) + \ln(x)}{\ln(x)} \, dx[/tex]

Grouping together terms in the numerator, we can write

[tex]\displaystyle -\int_0^1 \frac{1+x^2}{1+x^2+x^4} \frac{\ln(x^{2020}+1)-\ln(x+1)}{\ln(x)} \, dx + 2020 \int_0^1 \frac{1+x^2}{1+x^2+x^4} \, dx[/tex]

and the first term here will vanish with the other integral from the earlier split. So the original integral reduces to

[tex]\displaystyle \int_0^\infty \frac{1+x^2}{1+x^2+x^4} \frac{\ln(1-x+\cdots+x^{2020})}{\ln(x)} \, dx = 2020 \int_0^1 \frac{1+x^2}{1+x^2+x^4} \, dx[/tex]

Substituting [tex]x\to\frac1x[/tex] again shows this integral is the same over (0, 1) as it is over (1, ∞), and since the integrand is even, we ultimately have

[tex]\displaystyle \int_0^\infty \frac{1+x^2}{1+x^2+x^4} \frac{\ln(1-x+\cdots+x^{2020})}{\ln(x)} \, dx = 2020 \int_0^1 \frac{1+x^2}{1+x^2+x^4} \, dx \\\\ = 1010 \int_0^\infty \frac{1+x^2}{1+x^2+x^4} \, dx \\\\ = 505 \int_{-\infty}^\infty \frac{1+x^2}{1+x^2+x^4} \, dx[/tex]

We can neatly handle the remaining integral with complex residues. Consider the contour integral

[tex]\displaystyle \int_\gamma \frac{1+z^2}{1+z^2+z^4} \, dz[/tex]

where γ is a semicircle with radius R centered at the origin, such that Im(z) ≥ 0, and the diameter corresponds to the interval [-R, R]. It's easy to show the integral over the semicircular arc vanishes as R → ∞. By the residue theorem,

[tex]\displaystyle \int_{-\infty}^\infty \frac{1+x^2}{1+x^2+x^4}\, dx = 2\pi i \sum_\zeta \mathrm{Res}\left(\frac{1+z^2}{1+z^2+z^4}, z=\zeta\right)[/tex]

where [tex]\zeta[/tex] denotes the roots of [tex]1+z^2+z^4[/tex] that lie in the interior of γ; these are [tex]\zeta=\pm\frac12+\frac{i\sqrt3}2[/tex]. Compute the residues there, and we find

[tex]\displaystyle \int_{-\infty}^\infty \frac{1+x^2}{1+x^2+x^4} \, dx = \frac{2\pi}{\sqrt3}[/tex]

and so the original integral's value is

[tex]505 \times \dfrac{2\pi}{\sqrt3} = \boxed{\dfrac{1010\pi}{\sqrt3}}[/tex]

Answer:

ok so we have 10 gallons left and we get 40 miles per gallon so this is just multiplycaiton

10*40=400

so you can get 400 more miles

Hope This Helps!!!

Answer:

34496

Step-by-step explanation:

i dont know how 2 explain this but you have to do the traditional way of multiplying PLS MARK BRAINLIEST

Replace x with π/2 - x to get the equivalent integral

[tex]\displaystyle \int_{-\frac\pi2}^{\frac\pi2} \cos(\cot(x) - \tan(x)) \, dx[/tex]

but the integrand is even, so this is really just

[tex]\displaystyle 2 \int_0^{\frac\pi2} \cos(\cot(x) - \tan(x)) \, dx[/tex]

Substitute x = 1/2 arccot(u/2), which transforms the integral to

[tex]\displaystyle 2 \int_{-\infty}^\infty \frac{\cos(u)}{u^2+4} \, du[/tex]

There are lots of ways to compute this. What I did was to consider the complex contour integral

[tex]\displaystyle \int_\gamma \frac{e^{iz}}{z^2+4} \, dz[/tex]

where γ is a semicircle in the complex plane with its diameter joining (-R, 0) and (R, 0) on the real axis. A bound for the integral over the arc of the circle is estimated to be

[tex]\displaystyle \left|\int_{z=Re^{i0}}^{z=Re^{i\pi}} f(z) \, dz\right| \le \frac{\pi R}{|R^2-4|}[/tex]

which vanishes as R goes to ∞. Then by the residue theorem, we have in the limit

[tex]\displaystyle \int_{-\infty}^\infty \frac{\cos(x)}{x^2+4} \, dx = 2\pi i {} \mathrm{Res}\left(\frac{e^{iz}}{z^2+4},z=2i\right) = \frac\pi{2e^2}[/tex]

and it follows that

[tex]\displaystyle \int_0^\pi \cos(\cot(x)-\tan(x)) \, dx = \boxed{\frac\pi{e^2}}[/tex]

Answer:

Formula

Volume of a rectangular prism = width × length × height

Bottom Tier

volume = 12 × 18 × 8

            = 1,728 cm³

Middle Tier

volume = 8 × 12 × 6

            = 576 cm³

Top Tier

volume = 4 × 4 × 4

            = 64 cm³

Entire Cake

Volume = bottom tier + middle tier + top tier

             = 1728 + 576 + 64

             = 2,368 cm³

Answer:

A on edge

Step-by-step explanation:

:)

A triangle is a three-edged polygon with three vertices. The length of QS is equal to 17 units.

A triangle is a three-edged polygon with three vertices. It is a fundamental form in geometry. The sum of all the angles of a triangle is always equal to 180°.

Given that the Line l is a perpendicular bisector of line segment RQ and bisects it at point T. Therefore, the length of the two lines is,

RT = QT

Now, in ΔRST and ΔQST,

RT ≅ QT

ST ≅ ST {Common side}

Now, since the two triangles are the right-angled triangle, therefore, the two triangles are congruent. Thus, we can write,

RS = SQ

Given that the Line segment, RS is 3x+2. Line segment SQ is 5x-8.

RS = SQ

3x + 2 = 5x - 8

2 + 8 = 5x - 3x

10 = 2x

x = 5

QS = 5x - 8

     = 5(5) - 8

     = 25 - 8

     = 17 units

Hence, the length of QS is equal to 17 units.

Learn more about Triangle here:

https://brainly.com/question/2773823

#SPJ6

Hey there!

We're given 2 points that the line intersects, so we can easily determine the line's slope.

This is the formula:

[tex]\bold{\displaystyle\frac{y2-y1}{x2-x1} }[/tex]

[tex]\bold{\displaystyle\frac{5-(-6)}{-4-(-9)}}[/tex]

[tex]\bold{\displaystyle\frac{5+6}{-4+9}}[/tex]

[tex]\bold{\displaystyle\frac{11}{5}}[/tex]

Hence, the answer is

[tex]\boxed{\boxed{\bold{\frac{11}{5} }}}[/tex]

Hope everything is clear.

Let me know if you have any other questions!

#LearningDoesn'tEnd

:-)

[tex]\rule{200}{2}[/tex]

the ans for this q would be 5/27

Step-by-step explanation:

15 = 3/5 x = 3x/5

75 = 3x

x = 25 ounces