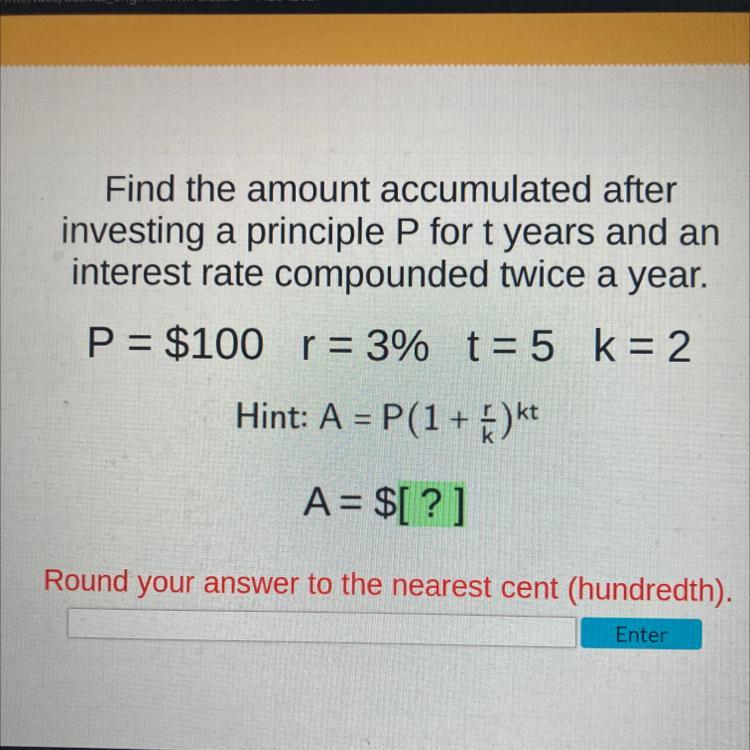
Find the amount accumulated after
investing a principle P for t years and an
interest rate compounded twice a year.
P = $100 r = 3% t = 5
k=2
Hint: A = P(1 + E) kt
A = $[?]
Answers
Answer:
A= $116.05
Step-by-step explanation:
A=P(1+E)kt
A=100(1+0.03/2)^10
A= $116.05
Related Questions
The table below shows the numbers of two to five bedroom houses in the Belmont Neighborhood. What is the mean number of bedrooms in a house in this neighborhood?
Number of bedrooms Frequency
2 7
3 35
4 56
5 27
Answers
The mean number of bedrooms in a house in this neighborhood will be: 3.824.
How to determine the mean number of bedroomsIn order to find the mean number of bedrooms in a house in the Belmont Neighborhood, we need to calculate the weighted mean by multiplying each number of bedrooms by their respective frequency.
Next, we will add the products, and then divide by the total frequency. So we can start as follows:
(2 * 7) + (3 * 35) + (4 * 56) + (5 * 27) = 14 + 105 + 224 + 135 = 478
Total frequency = 7 + 35 + 56 + 27 = 125
Therefore, the mean number of bedrooms = 478 / 125 approximately 3.824
Learn more about weighted mean here:
https://brainly.com/question/23902866
#SPJ1
f(x) = x2 + 4; interval [0, 5]; n = 5; use left endpoints
Answers
Therefore, the Left Riemann Sum for this function, interval, and number of subintervals is 50.
The left endpoint rule is what?The top-left corner of these rectangles touched the y=f(x) curve. In other words, the value of f at the subinterval's left endpoint determined the height of the rectangle over that subinterval. This technique is called the left-endpoint estimate because of this.
With left endpoints and n = 5 subintervals, we may use the Left Riemann Sum formula to approximate the area under the curve of f(x) = x2 + 4 over the range [0, 5]:
Left Riemann Sum = ∑[i=1 to n] f(x_i-1) Δx
In this case, a = 0, b = 5, n = 5, and we will use the left endpoints, so:
Δx = (5 - 0)/5 = 1
Using the left endpoints, the subintervals and their left endpoints are:
[0,1],[1,2],[2,3],[3,4],[4,5]
[tex]so,\; x_0 = 0, x_1 = 1, x_2 = 2, x_3 = 3, x_4 = 4.[/tex]
Now we can calculate the Left Riemann Sum:
Left Riemann Sum= [tex]f(x_0)\Delta x + f(x_1)\Deltax + f(x_2)\Deltax + f(x_3)\Deltax + f(x_4)\Deltax[/tex]
= f(0)×1+f(1)×1+f(2)×1+f(3)×1 + f(4)×1
= 4+5+8+13+20
= 50
To know more about the function visit:-
brainly.com/question/12431044
#SPJ1
4x+3y=-23and-x+y=-8 algebra elimination
Answers
I hope this helps you.
you can check your ans. by sub. in the value of x and y into both the equation which must give you the same answer.
Convert the following General Form Equations into Standard Form and then find the center and radius
Answers
Answer:
good luck
Step-by-step explanation:
Find the area of a shaded region
Answers
Answer:
40 cm²
Step-by-step explanation:
you have to multiply the top of the triangle by the base(40) which is 80 then you divide by two since it's a triangle then you get 40 again since your finding the area you have to put the 2 above the cm
Landon and Maria are meeting at the library to work on their history project. Maria walks 9 blocks east and 3 blocks north to get to the library from her house. Landon walks 5 blocks south and 7 blocks west to get to the library from his house. The map below shows the location of the library and Landon's and Maria's houses. To the nearest block, how far is Landon's house from Maria's house if Maria could walk in a straight line?
Answers
To the nearest block, Landon's house is at distance of 12 blocks away from Maria's house if Maria could walk in a straight line.
What is Pythagoras theorem?A basic mathematical theorem relating to the sides of a right-angled triangle is known as Pythagoras' theorem. The square of the length of the hypotenuse, the side that faces the right angle, is said to be equal to the sum of the squares of the lengths of the other two sides, known as the legs, in a right triangle.
This can be written in mathematical notation as:
c² = a² + b²
where c is the length of the hypotenuse, and a and b are the lengths of the legs of the right triangle.
In this case, we can consider the straight line between Maria's house and Landon's house as the hypotenuse of a right triangle, with the distances they walked as the other two sides. We can use the distance formula to find the lengths of those sides:
Distance walked by Maria = √(9² + 3²) = √90 ≈ 9.49 blocks
Distance walked by Landon = √(5² + 7²) = √74 ≈ 8.60 blocks
Now we can use the Pythagorean theorem to find the distance between their houses:
Distance between houses = √(9.49² + 8.60²) ≈ 12.46 blocks
To know more about Pythagoras' theorem, visit:
https://brainly.com/question/21926466
#SPJ1
Given the figure below .find X and Y to three significant digits.Write your answer in the answer box provided below
Answers
Check the picture below.
Make sure your calculator is in Degree mode.
[tex]\cos(25^o )=\cfrac{\stackrel{adjacent}{x}}{\underset{hypotenuse}{12}}\implies 12\cos(25^o)=x\implies \boxed{10.876\approx x} \\\\[-0.35em] ~\dotfill\\\\ \sin(25^o )=\cfrac{\stackrel{opposite}{z}}{\underset{hypotenuse}{12}}\implies 12\sin(25^o)=z \\\\[-0.35em] ~\dotfill\\\\ \sin(50^o )=\cfrac{\stackrel{opposite}{z}}{\underset{hypotenuse}{y}}\implies y=\cfrac{z}{\sin(50^o)}\implies y=\cfrac{12\sin(25^o)}{\sin(50^o)}\implies \boxed{y\approx 6.62}[/tex]
The expression below is equal to -20g plus a constant term. -8(2.5g-4.25)+5.25 What is the value of the constant term?
Answers
Answer:
We can simplify the expression -8(2.5g-4.25)+5.25 by distributing the -8:
-8(2.5g-4.25)+5.25 = -20g + 34 + 5.25
Simplifying further, we can combine the constant terms:
-8(2.5g-4.25)+5.25 = -20g + 39.25
So the value of the constant term is 39.25.
how can you tell if a quotient is less than 1'.
Answers
Answer:
Step-by-step explanation:
Okay so when a smaller number is divided by a larger number, the quotient is less than 1. Hope this helps Bye sisters slay.
If you are performing a left-tailed z-statistic test with a sample size n = 85 and a 0.01 significance level, what is the critical value?
If your calculated value of the z-statistic is z = −1.95, what is your conclusion about the null hypothesis (reject the null hypothesis or fail to reject the null hypothesis)?
Answers
This null hypothesis prompt is actually straight forward. Note that where the above conditions exist, we must NOT reject the null hypothesis due to the values of the z-statistics and the significance level.
What is a nyll hypothesis?The idea that there is no influence on the population is known as the null hypothesis. We can reject the null hypothesis if the sample contains sufficient data to refute the assertion that there is no impact in the population (p≤α ).
So to determine whether or not to accept or reject the null hypothesis, we must determine dthe z-score.
To find the the critial value for a left-tailoed test, where the signifiance level is 0.01, note that we must find the z-score that leaves 1% of the area in the left tail.
Using the standard distribution table, the z-score for 0.01 left tail is about -2.33.
Since
z-satistics which is equal to -1.95 (as given) is greater than the z-score, we must NOT reject the null hypothesis.
Learn more about null hypothesis:
https://brainly.com/question/28920252
#SPJ1
Find the critical value t
Answers
The answer of the given question based on the Critical value is , , the critical value to for the confidence level c = 0.99 and sample size n = 22 is 2.819.
What is Critical value?In statistics, the critical value is a value that is used to determine whether to reject the null hypothesis in a hypothesis test. It is based on the chosen level of significance, which is the maximum probability of making a Type I error (rejecting a true null hypothesis). The critical value is determined by the sampling distribution of the test statistic, which is often a t-statistic or z-statistic, depending on the test and the characteristics of the population being studied.
To find the critical value t for a 99% confidence level and a sample size of 22, we need to use a t-distribution table or a calculator.
Using a t-distribution table with 21 degrees of freedom (n-1), we find that the critical value for a 99% confidence level is approximately 2.819.
Therefore, critical value for confidence level c = 0.99 and sample size n = 22 is 2.819 (rounded to nearest thousandth).
To know more about Null hypothesis visit:
https://brainly.com/question/30404845
#SPJ1
Triangle PQR is rotated 180 degrees clockwise about the origin to produce the image Triangle P’Q’R’. Which of the following statements is TRUE abóyate Triangle P’Q’R’.
Answers
Answer:
Step-by-step explanation:
We get triangle PQR by plotting the point P (1, 4), Q (3, 1), R (2, -1) on the graph paper when rotated through 180° about the origin. The new position of the point is: P (1, 4) → P' (-1, -4) Q (3, 1) → Q' (-3, -1) R (2, -1) → R' (-2, 1) Thus, the new position of ∆PQR is ∆P’Q’R’.
A cone with a radius of 4 inches and a slant height of 10 inches is shown.
What is the surface area of the cone, in square inches?
62.8
125.7
150.8
O 175.9
4 in.
10 in.
Answers
Answer:
167.6190 approximately 167.62 inches
Step-by-step explanation:
formula for surface area of a cone is 1/3πr×r×slant height. How this helps.
Use the information below to determine the new coordinates of the image under the given translation.
Triangle ABC with vertices
A(0, 7), B(7, 3),
and C(1, 4): (x, y) → (x − 3, y - 4)
Answers
The new triangle A'B'C' after the translation is A'(-3, 3), B'(4, -1), and C'(-2, 0).
What is translation?A shape is translated when it is moved up, down, left or right without turning. They are congruent if the translated shapes (or the image) seem to be the same size as the original shapes. They have only changed their direction or directions.
To perform the given translation (x, y) → (x − 3, y - 4) on the triangle ABC with vertices A(0, 7), B(7, 3), and C(1, 4), we need to apply the same transformation to each vertex of the triangle and obtain their new coordinates.
For vertex A(0, 7), the transformation gives:
(x, y) → (x − 3, y - 4)
(0, 7) → (0 - 3, 7 - 4)
The new coordinates of A are (-3, 3).
For vertex B(7, 3), the transformation gives:
(x, y) → (x − 3, y - 4)
(7, 3) → (7 - 3, 3 - 4)
The new coordinates of B are (4, -1).
For vertex C(1, 4), the transformation gives:
(x, y) → (x − 3, y - 4)
(1, 4) → (1 - 3, 4 - 4)
The new coordinates of C are (-2, 0).
Therefore, the new triangle A'B'C' after the translation is A'(-3, 3), B'(4, -1), and C'(-2, 0).
Learn more about translation on:
https://brainly.com/question/1046778
#SPJ1
i have 60 square feet of paper to wrap a box in the shape of a right rectangular prism the height of the box is 1 2/3 feet the width is 4 feet and the length is 5 1/2 feet what percent of the box will remain unwrapped if i use all the paper i has available
Answers
The percent of the box that will remain unwrapped by the 60 square feet of paper is about 20.70%
What is a percentage?A percentage is a proportion of a number expressed as fraction of a 100
The dimensions in mixed fractions can be expressed as follows;
Height of the box = 1 2/3 feet = 5/3 feet
Width of the box = 4 feet
Length of the box = 5 1/2 feet = 11/2 feet
Surface area of the box, A = 2 × (5/3) × 4 + 2 × 4 × 11/2 + 2 × (5/3) × 11/2 = 227/3 = 75 2/3 square inches
The percent of the box that will remain unwrapped is therefore;
Percentage = 60/(227/3) × 100 ≈ 79.3%
The percentage of the box that will remain unwrapped is about (100 - 79.3)% = 20.70%
Learn more on the percentages here: https://brainly.com/question/11642822
#SPJ1
Can someone help me with this problem, and explain how to solve it?
Answers
Thus, the height of flagpole from the ground is found as 23.34 feet.
Explain about the angle of elevation:The measurement of the angle between a person's eyes' line of sight to anything above and the horizontal line is known as the angle of elevation.
The movement of the observer's eyes determines the elevation angle. The angle of elevation is the angle formed by the line of sight and the horizontal line when a viewer is looking up at an object.
Given data:
height of boy = 5 ftDistance of boy from flagpole = 30 ft angle of elevation = 35 degreesLet the height of pole above the boy be 'h'feet.Using the trigonometric ratios in the right triangle ABE.
tan 35 = h / 30
h = 30* tan(35)
h = 18.34
Height of flagpole = 18.34 + 5 = 23.34 feet
Thus, the height of the flagpole from the ground is found as 23.34 feet.
Know more about the angle of elevation:
https://brainly.com/question/26356439
#SPJ1
1. All numbered streets runs parallel to each other. Both 3rd and 4th Streets are intersected by King Ave. as shown:
(a) Suppose a car is traveling east on 4th Street and turns onto King Avenue heading northeast. What is the measure of the angle created by the car's turning? Explain your answer.
(b) Suppose a car is traveling southwest on King Avenue and turns left onto 3rd Street. What is the measure of the angle created by the car's turning? Explain your answer.
(c) Suppose a car is traveling northeast on King Avenue and turns right onto 3rd Street. What is the measure of the angle created by the car's turning? Explain your answer.
Answers
When the car travels east on 4th Street and turns onto King Avenue heading northeast, the angle created by the car's turning is a 45-degree angle.
When the car travels southwest on King Avenue and turns left onto 3rd Street, the angle created by the car's turning is a 135-degree angle.
When the car travels northeast on King Avenue and turns right onto 3rd Street, the angle created by the car's turning is a 45-degree angle.
How to get the Angle?(a) When the car travels east on 4th Street and turns onto King Avenue heading northeast, the angle created by the car's turning is a 45-degree angle. This is because the intersection of 4th Street and King Avenue creates a right angle, and the car turns northeast, creating another 45-degree angle with the horizontal 4th Street.
(b) When the car travels southwest on King Avenue and turns left onto 3rd Street, the angle created by the car's turning is a 135-degree angle. This is because the intersection of King Avenue and 3rd Street creates a right angle, and the car turns left, creating an additional 90-degree angle. Therefore, the total angle is 90 degrees + 45 degrees = 135 degrees.
(c) When the car travels northeast on King Avenue and turns right onto 3rd Street, the angle created by the car's turning is a 45-degree angle. This is because the intersection of King Avenue and 3rd Street creates a right angle, and the car turns right, creating another 45-degree angle with the horizontal 3rd Street.
Learn more about right angle here: https://brainly.com/question/29869536
#SPJ1
Is a measure of 30 inches "far away" from a mean of 15 inches? As someone with knowledge of statistics, you answer "it depends" and request the standard deviation of the underlying data.
(a) Suppose the data come from a sample whose standard deviation is 3 inches. How many standard deviations is inches from 15 inches?
(b) Is 30 inches far away from a mean of 15 inches?
(c) Suppose the standard deviation of the underlying data is 10 inches. Is 30 inches far away from a mean of 15 inches?
Answers
Hence, 30 inches deviate by 1.5 standard deviations from the 15-inch mean as the underlying data's standard deviation is 10 inches.
what is standard deviation ?The standard deviation of statistics is a measurement of how much a group of data values deviate from their mean (average) value. While a significant standard deviation suggests that the pieces of information are dispersed throughout a broader range of values, a low standard deviation suggests that the data points are generally close to the mean.
given
a) If the sample's standard deviation is 3 inches, then the number of standard deviations from the mean of 15 inches that are 30 inches is:
z = (30 - 15) / 3 = 5
b) The context of the data and the standard deviation will determine whether 30 inches is distant from a mean of 15 inches.
A departure of 15 inches from the mean, meanwhile, can be viewed as being significantly off the mean if the standard deviation is minimal.
c) If the underlying data's standard deviation is 10 inches, then the number of standard deviations from the mean of 15 inches that are 30 inches is:
z = (30 - 15) / 10 = 1.5
Hence, 30 inches deviate by 1.5 standard deviations from the 15-inch mean as the underlying data's standard deviation is 10 inches.
To know more about standard deviation visit:
https://brainly.com/question/23907081
#SPJ1
Pls help with this question
Answers
The plane degrees off course is 6.79 degrees off at a speed of 541. 92 km / hr relative to the ground.
How to find the degrees off course ?To find the airplane's course deviation and its ground speed, we need to first determine the velocity vectors of the airplane and the wind.
W x = W x cos(225) = 90 x cos(225) = -63.64 km/hr
Wy = W x sin(225) = 90 x sin(225) = -63.64 km/hr
Magnitude: |R| = sqrt(Rx^2 + Ry^2) = √(536.36^2 + (-63.64)^2) = 541.92 km/hr
Direction: θ = arctan(Ry / Rx) ≈ arctan (-63.64 / 536.36) = -6.79 degrees
So, the airplane is 6.79 degrees off course, and its ground speed is 541.92 km/hr.
Find out more on airplanes at https://brainly.com/question/10922845
#SPJ1
Convert to polar form
Y=x^2-64/16
Answers
To convert the expression, Y=x^2-64/16 into the polar form, we would have: r = 2 / √(sin^2θ + cos^2θ).
How to convert into the polar formThe polar form of a mathematical expression is a way of representing complex numbers using their magnitude and angle.
For a complex number z = a + bi, where a and b are real numbers, its polar form is given by z = r(cosθ + i sinθ), where r is the magnitude of z and θ is the angle that z makes with the positive real axis.
So, for the given expression, the polar form can be converted this way:
r^2 sin^2θ = r cos^2θ - 4
r^2(sin^2θ + cos^2θ) = 4
r = 2 / √(sin^2θ + cos^2θ)
Therefore, the polar form is r = 2 / √(sin^2θ + cos^2θ).
Learn more about the polar form of a mathematical expression here:
https://brainly.com/question/28967624
#SPJ1
2298/24 what is the quotient step by step
Answers
2298 divided by 24 using long division shows what is the quotient and remainder while 2298 divided by 24 and the complete steps for how to find it.
Question:
2298/24 = (?)
what is 2298 divided by 24?
Answer:the quotient is 95 and the remainder is 18 when 2298 is divided by 24.
The histograms display the frequency of temperatures in two different locations in a 30-day period.
A graph with the x-axis labeled Temperature in Degrees, with intervals 60 to 69, 70 to 79, 80 to 89, 90 to 99, 100 to 109, 110 to 119. The y-axis is labeled Frequency and begins at 0 with tick marks every one unit up to 14. A shaded bar stops at 10 above 60 to 69, at 9 above 70 to 79, at 5 above 80 to 89, at 4 above 90 to 99, and at 2 above 100 to 109. There is no shaded bar above 110 to 119. The graph is titled Temps in Sunny Town.
A graph with the x-axis labeled Temperature in Degrees, with intervals 60 to 69, 70 to 79, 80 to 89, 90 to 99, 100 to 109, 110 to 119. The y-axis is labeled Frequency and begins at 0 with tick marks every one unit up to 14. There is no shaded bar above 60 to 69. A shaded bar stops at 4 above 70 to 79, at 4 above 80 to 89, at 6 above 90 to 99, at 6 above 100 to 109 and at 10 above 110 to 119. The graph is titled Temps in Beach Town.
When comparing the data, which measure of center should be used to determine which location typically has the cooler temperature?
Median, because Sunny Town is symmetric
Mean, because Sunny Town is skewed
Median, because Beach Town is skewed
Mean, because Beach Town is symmetric
Answers
Answer:
Whats the question?
Step-by-step explanation:
What number increased by 3.5% of itself equals 621?
Answers
Answer:
Step-by-step explanation:
We need to find a number which increased by 3.5 of itself equals 621.
Let that number be x.
x increased by 3.5 of itself equals 621 implies x+ 3.5x = 621.
x(1+3.5) = 621
x(4.5) = 621
x = 621/4.5
x = 138
Therefore the required number is 138.
Answer: 138
Step-by-step explanation:
We need to find a number which increased by 3.5 of itself equals 621.
Let that number be x.
x increased by 3.5 of itself equals 621 implies x+ 3.5x = 621.
x(1+3.5) = 621
x(4.5) = 621
x = 621/4.5
x = 138
Therefore the required number is 138.
If you horizontally…
Answers
Check the picture below.
so following the template below, a shift 2 units to the left, means C=2
[tex]G(x)=\sqrt{x+2}[/tex]
which of these is another way to write the function g(x)= 3x
Answers
Answer:
y=9 (3x/9-2) + 18=3x
Step-by-step explanation:
Which expression represents the phrase The sum of w and twenty
A. 20w
B. w + 20
C. w^20
D. 20 x w
Answers
^4√p7
in exponential form.
Answers
Answer:1111
Step-by-step explanation:
Brenda is fishing from a small boat. Her fishing hook is 12 feet below her, and a fish is swimming at the same depth as the hook, 16 feet away. How far away is Brenda from the fish?
Answers
Answer:
We can use the Pythagorean theorem to solve this problem. Let's call the distance Brenda is away from the fish "x". Then, we have a right triangle with legs of length 12 and x, and a hypotenuse of length 16. So:
x^2 + 12^2 = 16^2
Simplifying:
x^2 + 144 = 256
x^2 = 112
Taking the square root of both sides:
x ≈ 10.6 feet
Therefore, Brenda is approximately 10.6 feet away from the fish.
Please help will mark Brainliest
Answers
Step-by-step explanation:
5 cos (307) = 3
5 sin (307) = -4
just plot the point ( 3, -4 ) Done.
Answer:
The point is (3, -4) because 5 cos [307] = 3 and 5 sin [307] = -4
Hello, I am confused on how to answer this question.
Answers
Answer: x=14
Step-by-step explanation:
cb=10.
ac=14
The value of X will be 14.
This is a simple mathematics problem that can be solved by using ratios.
Given, AC : BC : AB = 7 : 5 : 8
AC = X ; BC = 10 ; AB = X + 2
AC/ BC = X/ 10 = 7/5 (Ratios can also be represented as fractions)
X/10 = 7/5
On Transposing,
5X = 70
X = 70/5
X = 14
Alternatively,
BC/AB = 5/8 = 10/ (X + 2)
5/8 = 10/ (X + 2)
On Transposing,
80 = 5X + 10
5X = 70
X = 14
To know more about Ratios,
https://brainly.com/question/29442975